Geometric Sequences
Finding Common Ratios
The yearly salary values described form a geometric sequence because they change by a constant factor each year. Each term of a geometric sequence increases or decreases by a constant factor called the common ratio. The sequence below is an example of a geometric sequence because each term increases by a constant factor of 6. Multiplying any term of the sequence by the common ratio 6 generates the subsequent term.
A General Note: Definition of a Geometric Sequence
A geometric sequence is one in which any term divided by the previous term is a constant. This constant is called the common ratio of the sequence. The common ratio can be found by dividing any term in the sequence by the previous term. If is the initial term of a geometric sequence and is the common ratio, the sequence will be.
How To: Given a set of numbers, determine if they represent a geometric sequence.
- Divide each term by the previous term.
- Compare the quotients. If they are the same, a common ratio exists and the sequence is geometric.
Example 1: Finding Common Ratios
Is the sequence geometric? If so, find the common ratio.- .
- .
Solution
Divide each term by the previous term to determine whether a common ratio exists.- The sequence is geometric because there is a common ratio. The common ratio is 2.
- The sequence is not geometric because there is not a common ratio.
Analysis of the Solution
The graph of each sequence is shown in Figure 1. It seems from the graphs that both (a) and (b) appear have the form of the graph of an exponential function in this viewing window. However, we know that (a) is geometric and so this interpretation holds, but (b) is not.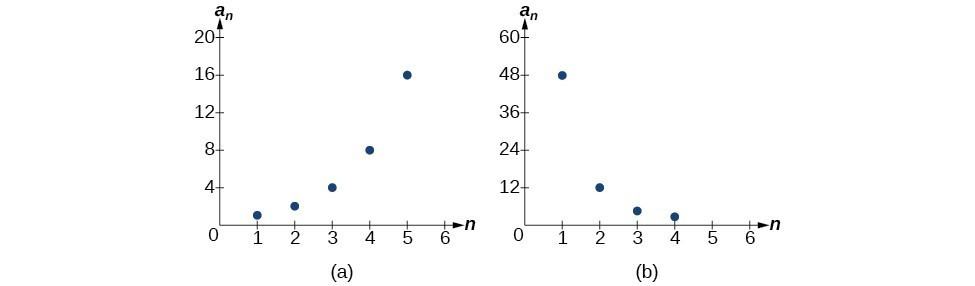 Figure 1
Figure 1Q & A
If you are told that a sequence is geometric, do you have to divide every term by the previous term to find the common ratio?
No. If you know that the sequence is geometric, you can choose any one term in the sequence and divide it by the previous term to find the common ratio.Try It 1
Is the sequence geometric? If so, find the common ratio..
Solution
Try It 2
Is the sequence geometric? If so, find the common ratio..
Solution
Writing Terms of Geometric Sequences
Now that we can identify a geometric sequence, we will learn how to find the terms of a geometric sequence if we are given the first term and the common ratio. The terms of a geometric sequence can be found by beginning with the first term and multiplying by the common ratio repeatedly. For instance, if the first term of a geometric sequence is and the common ratio is , we can find subsequent terms by multiplying to get then multiplying the result to get and so on.
The first four terms are .
How To: Given the first term and the common factor, find the first four terms of a geometric sequence.
- Multiply the initial term, , by the common ratio to find the next term, .
- Repeat the process, using to find and then to find until all four terms have been identified.
- Write the terms separated by commons within brackets.
Example 2: Writing the Terms of a Geometric Sequence
List the first four terms of the geometric sequence with and .Solution
Multiply by to find . Repeat the process, using to find , and so on.
The first four terms are .
Try It 3
List the first five terms of the geometric sequence with and . SolutionUsing Recursive Formulas for Geometric Sequences
A recursive formula allows us to find any term of a geometric sequence by using the previous term. Each term is the product of the common ratio and the previous term. For example, suppose the common ratio is 9. Then each term is nine times the previous term. As with any recursive formula, the initial term must be given.A General Note: Recursive Formula for a Geometric Sequence
The recursive formula for a geometric sequence with common ratio and first term isHow To: Given the first several terms of a geometric sequence, write its recursive formula.
- State the initial term.
- Find the common ratio by dividing any term by the preceding term.
- Substitute the common ratio into the recursive formula for a geometric sequence.
Example 3: Using Recursive Formulas for Geometric Sequences
Write a recursive formula for the following geometric sequence.Solution
The first term is given as 6. The common ratio can be found by dividing the second term by the first term.
Substitute the common ratio into the recursive formula for geometric sequences and define .
Analysis of the Solution
The sequence of data points follows an exponential pattern. The common ratio is also the base of an exponential function as shown in Figure 2.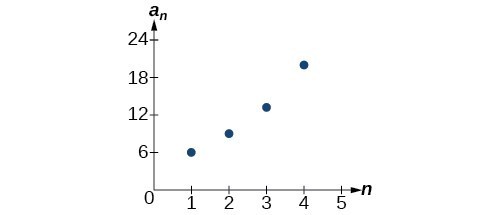 Figure 2
Figure 2Q & A
Do we have to divide the second term by the first term to find the common ratio?
No. We can divide any term in the sequence by the previous term. It is, however, most common to divide the second term by the first term because it is often the easiest method of finding the common ratio.Try It 4
Write a recursive formula for the following geometric sequence.
Solution
Using Explicit Formulas for Geometric Sequences
Because a geometric sequence is an exponential function whose domain is the set of positive integers, and the common ratio is the base of the function, we can write explicit formulas that allow us to find particular terms.
Let’s take a look at the sequence . This is a geometric sequence with a common ratio of 2 and an exponential function with a base of 2. An explicit formula for this sequence is
The graph of the sequence is shown in Figure 3.
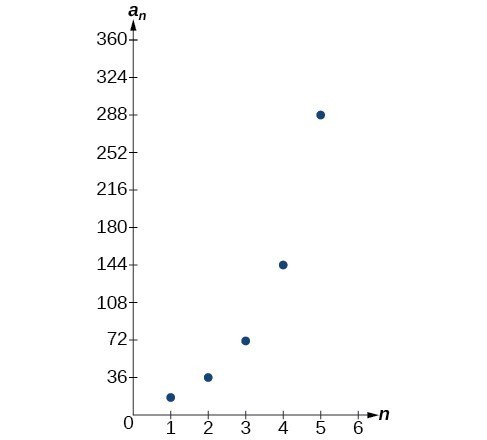 Figure 3
Figure 3A General Note: Explicit Formula for a Geometric Sequence
The nth term of a geometric sequence is given by the explicit formula:Example 4: Writing Terms of Geometric Sequences Using the Explicit Formula
Given a geometric sequence with and , find .Solution
The sequence can be written in terms of the initial term and the common ratio ..
Find the common ratio using the given fourth term.
Find the second term by multiplying the first term by the common ratio.
Analysis of the Solution
The common ratio is multiplied by the first term once to find the second term, twice to find the third term, three times to find the fourth term, and so on. The tenth term could be found by multiplying the first term by the common ratio nine times or by multiplying by the common ratio raised to the ninth power.Try It 5
Given a geometric sequence with and , find . SolutionExample 6: Writing an Explicit Formula for the nth Term of a Geometric Sequence
Write an explicit formula for the term of the following geometric sequence.Solution
The first term is 2. The common ratio can be found by dividing the second term by the first term.
The common ratio is 5. Substitute the common ratio and the first term of the sequence into the formula.
The graph of this sequence in Figure 4 shows an exponential pattern.
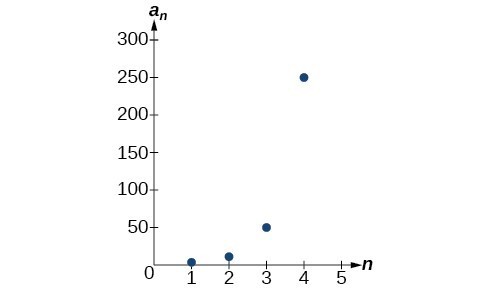 Figure 4
Figure 4Try It 6
Write an explicit formula for the following geometric sequence.Solution
Solving Application Problems with Geometric Sequences
In real-world scenarios involving arithmetic sequences, we may need to use an initial term of instead of . In these problems, we can alter the explicit formula slightly by using the following formula:Example 7: Solving Application Problems with Geometric Sequences
In 2013, the number of students in a small school is 284. It is estimated that the student population will increase by 4% each year.- Write a formula for the student population.
- Estimate the student population in 2020.
Solution
- The situation can be modeled by a geometric sequence with an initial term of 284. The student population will be 104% of the prior year, so the common ratio is 1.04.Let be the student population and be the number of years after 2013. Using the explicit formula for a geometric sequence we get
- We can find the number of years since 2013 by subtracting.
We are looking for the population after 7 years. We can substitute 7 for to estimate the population in 2020.The student population will be about 374 in 2020.
Try It 7
A business starts a new website. Initially the number of hits is 293 due to the curiosity factor. The business estimates the number of hits will increase by 2.6% per week.a. Write a formula for the number of hits.
b. Estimate the number of hits in 5 weeks.
Solution
Key Equations
| recursive formula for term of a geometric sequence | |
| explicit formula for term of a geometric sequence |
Key Concepts
- A geometric sequence is a sequence in which the ratio between any two consecutive terms is a constant.
- The constant ratio between two consecutive terms is called the common ratio.
- The common ratio can be found by dividing any term in the sequence by the previous term.
- The terms of a geometric sequence can be found by beginning with the first term and multiplying by the common ratio repeatedly.
- A recursive formula for a geometric sequence with common ratio is given by for .
- As with any recursive formula, the initial term of the sequence must be given.
- An explicit formula for a geometric sequence with common ratio is given by .
- In application problems, we sometimes alter the explicit formula slightly to .
Glossary
- common ratio
- the ratio between any two consecutive terms in a geometric sequence
- geometric sequence
- a sequence in which the ratio of a term to a previous term is a constant
Section Exercises
1. What is a geometric sequence? 2. How is the common ratio of a geometric sequence found? 3. What is the procedure for determining whether a sequence is geometric? 4. What is the difference between an arithmetic sequence and a geometric sequence? 5. Describe how exponential functions and geometric sequences are similar. How are they different? For the following exercises, find the common ratio for the geometric sequence. 6. . 7. . 8. . For the following exercises, determine whether the sequence is geometric. If so, find the common ratio. 9. . 10. . 11. . 12. . 13. . For the following exercises, write the first five terms of the geometric sequence, given the first term and common ratio. 14. 15. For the following exercises, write the first five terms of the geometric sequence, given any two terms. 16. 17. For the following exercises, find the specified term for the geometric sequence, given the first term and common ratio. 18. The first term is , and the common ratio is . Find the 5th term. 19. The first term is 16 and the common ratio is . Find the 4th term. For the following exercises, find the specified term for the geometric sequence, given the first four terms. 20. . Find . 21. . Find . For the following exercises, write the first five terms of the geometric sequence. 22. 23. For the following exercises, write a recursive formula for each geometric sequence. 24. 25. 26. 27. 28. 29. 30. 31. For the following exercises, write the first five terms of the geometric sequence. 32. 33. For the following exercises, write an explicit formula for each geometric sequence. 34. 35. 36. 37. 38. 39. 40. 41. For the following exercises, find the specified term for the geometric sequence given. 42. Let , . Find . 43. Let . Find . For the following exercises, find the number of terms in the given finite geometric sequence. 44. 45. For the following exercises, determine whether the graph shown represents a geometric sequence. 46. 47.
47.
 For the following exercises, use the information provided to graph the first five terms of the geometric sequence.
48.
49.
50.
51. Use recursive formulas to give two examples of geometric sequences whose 3rd terms are .
52. Use explicit formulas to give two examples of geometric sequences whose 7th terms are .
53. Find the 5th term of the geometric sequence .
54. Find the 7th term of the geometric sequence .
55. At which term does the sequence exceed
56. At which term does the sequence begin to have integer values?
57. For which term does the geometric sequence first have a non-integer value?
58. Use the recursive formula to write a geometric sequence whose common ratio is an integer. Show the first four terms, and then find the 10th term.
59. Use the explicit formula to write a geometric sequence whose common ratio is a decimal number between 0 and 1. Show the first 4 terms, and then find the 8th term.
60. Is it possible for a sequence to be both arithmetic and geometric? If so, give an example.
For the following exercises, use the information provided to graph the first five terms of the geometric sequence.
48.
49.
50.
51. Use recursive formulas to give two examples of geometric sequences whose 3rd terms are .
52. Use explicit formulas to give two examples of geometric sequences whose 7th terms are .
53. Find the 5th term of the geometric sequence .
54. Find the 7th term of the geometric sequence .
55. At which term does the sequence exceed
56. At which term does the sequence begin to have integer values?
57. For which term does the geometric sequence first have a non-integer value?
58. Use the recursive formula to write a geometric sequence whose common ratio is an integer. Show the first four terms, and then find the 10th term.
59. Use the explicit formula to write a geometric sequence whose common ratio is a decimal number between 0 and 1. Show the first 4 terms, and then find the 8th term.
60. Is it possible for a sequence to be both arithmetic and geometric? If so, give an example.